 |
The experience of the treatment of scoliosis leads us to consider two different cases. Below 25°, it is impossible to foresee the progression of the scoliosis, we are in a chaotic zone, above 25°, the progression is characterized by a curve well described by Mrs Duval Beaupère. |
 |
The first publication on chaos theory dates from my book published in 1986. A mathematical approach was presented in 2012. |
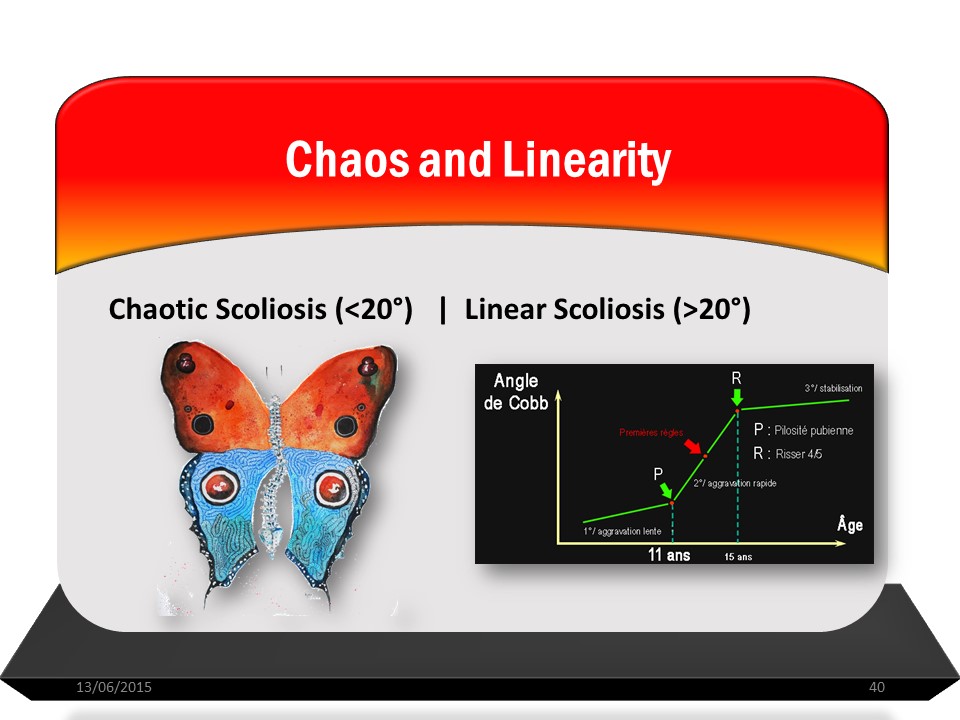 |
There are indeed 2 ways for scoliosis to progress. Linear scoliosis of more than 25 ° with the vicious circle during adolescence and chaotic scoliosis of less than 20 ° for which it is impossible to establish a progression prognosis. |
 |
We will study in more detail the characteristics of a chaotic system. |
In a non-chaotic system (such as a simple pendulum), changing the initial state slightly leads to only a slight change in the way the system develops. For example, if the simple pendulum started from a position slightly higher than the one shown here, it would move on an arc that is only slightly longer with a speed that is only slightly larger, but the overall trajectories would look almost the same. On the other hand, if you changed the initial position of the double pendulum slightly, it would lead to a trajectory that is completely different from the one shown here, and this is the most important property of a chaotic system: Any change (however small) in the initial state of the system leads to a completely different development of the system after some time. A scoliosis of more than 25 ° will always evolve according to the laws of Duval Beaupère. The results of the treatments are relatively homageneous. A scoliosis of less than 25 ° will evolve as the double pendulum and a small initial change may result in a totally different prognosis. This is fundamental for physiotherapy. |
|
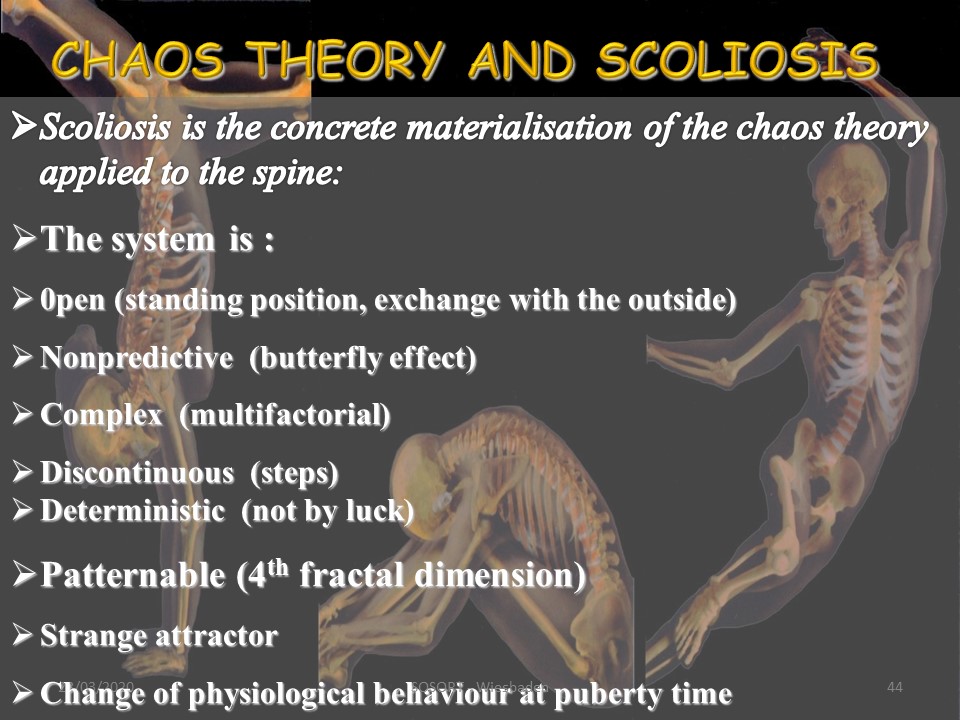 |
Scoliosis is the concrete materialisation of the chaos theory applied to the spine. The system is : 0pen (standing position, exchange with the outside), Non predictive (butterfly effect), Complex (multifactorial), Discontinuous (steps), Deterministic (not by luck), Patternable (4th fractal dimension), with a Strange attractor, and Change of physiological behaviour at puberty time. |
 |
The impossibility of predicting the evolution of scoliosis at this stage is the butterfly effect well described by Edward Lorentz: : « Does the flap of a butterfly's wings in Brazil, set up a tornado in Texas ?». |
 |
The apple falls according to the gravitation law of Newton, and we can foresee the place of the fall. The tree leaf falls according to the same laws, But we can not foresee the exact place of the fall, Because it is more sensitive to the wind. The unpredictibility of such phenomenas leads to an extreme sensibility to initial conditions, it is the deterministic chaos. |
 |
If a snowflake may cause an avalanche while predicting by calculation will tell us exactly what precise snowflake intercept for the avalanche does not happen! In fact: a supercritical state remains a supercritical state, and remove this particular snowflake would only allow another to replace it in this function. Nothing would be solved (accepted view today). |
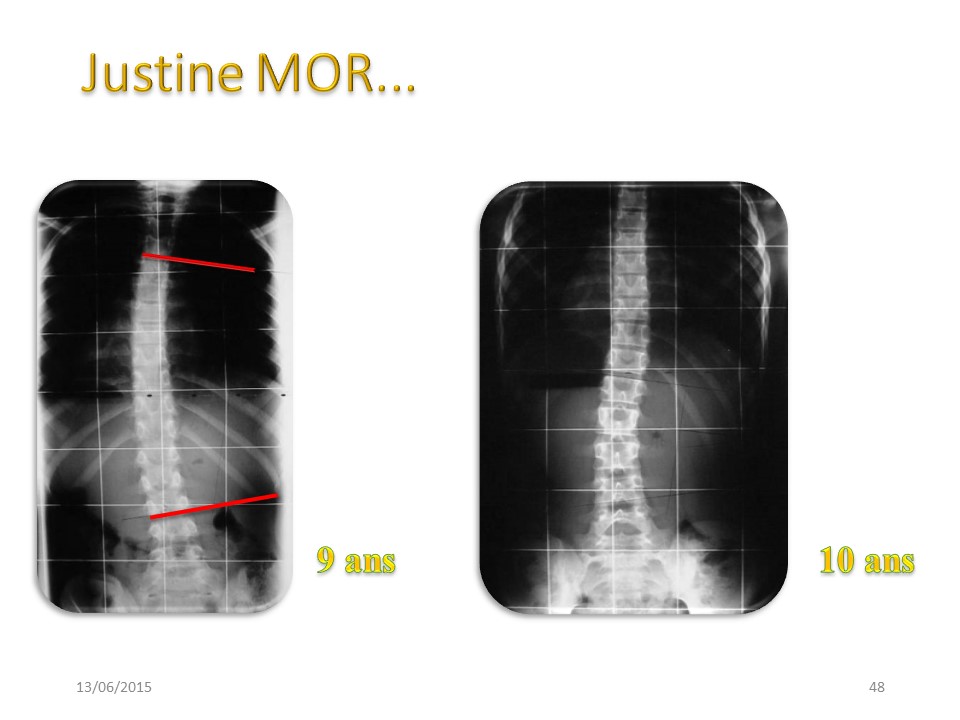 |
We frequently see in consultation scoliosis below 20 ° to be completely corrected at the next check without any special treatment. This is why it is very difficult to assert the effectiveness of physiotherapy for this type of scoliosis. |
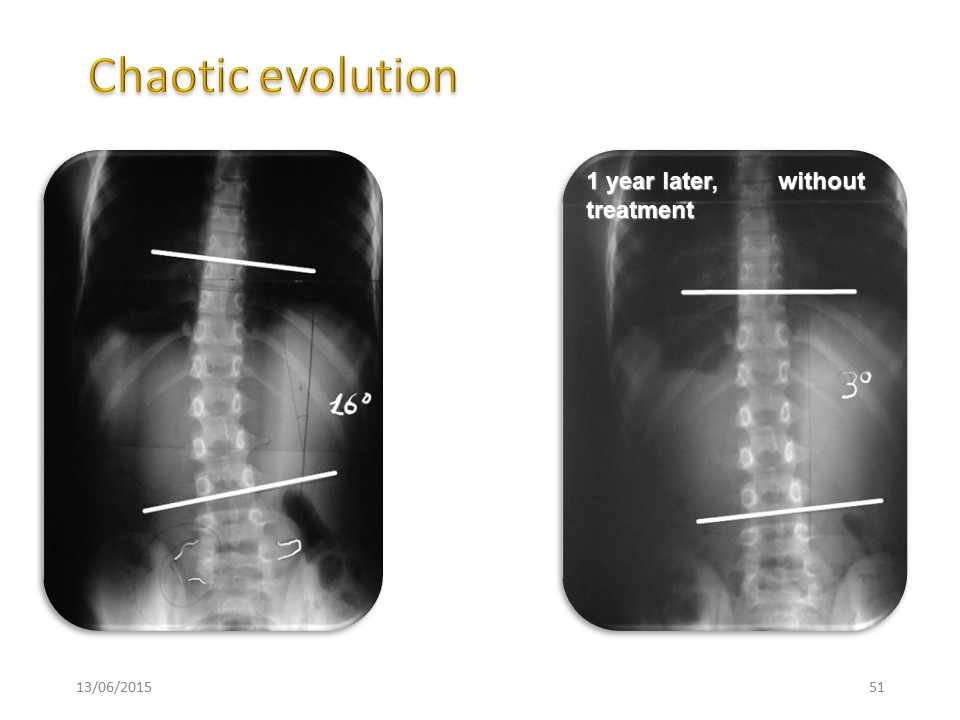 |
Another example of chaotic evolution without any treatment. |
 |
The existence of a strange attractor has been described by a French mathematician. The average of all the movements of the spine during the day, is no longer a straight column, but a curved column. |
 |
A chaotic system is always a complex system like the double pendulum. |
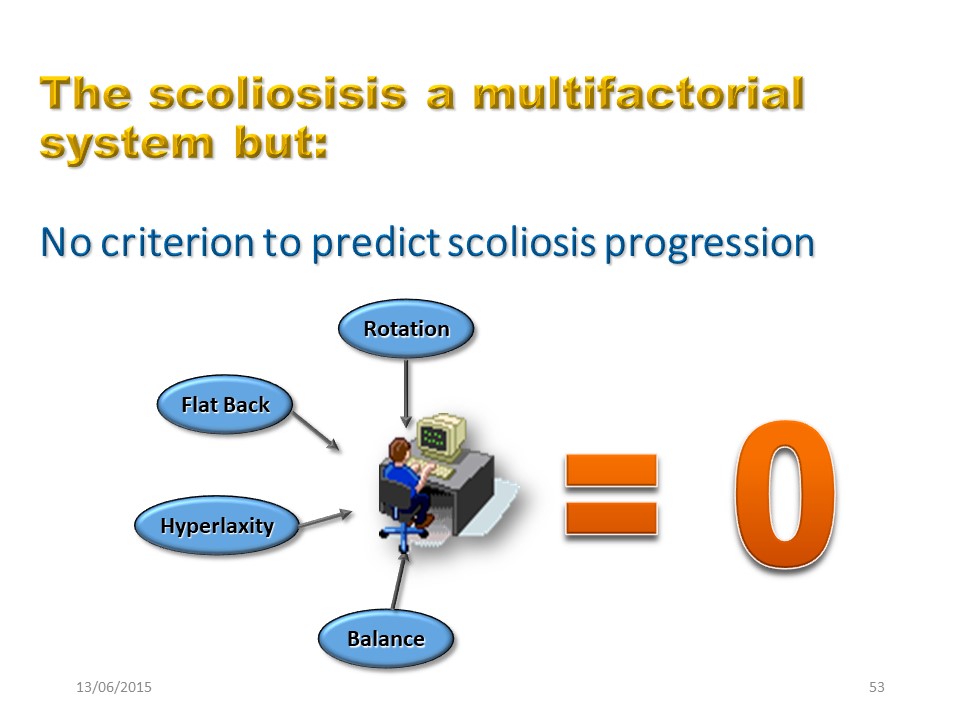 |
The etiology of the scoliosis is multifactorial and complex. When we gather in a computer the diferent characteristic of a progressive scoliosis, the flat back, the rotation, the hyperlaxity and the balance disturbance… with none of those characteristics we can predict the evolution of the scoliosis. Only an angle above 25° is a characteristic of progressive scoliosis, but we are not anymore in a chaos phase. |
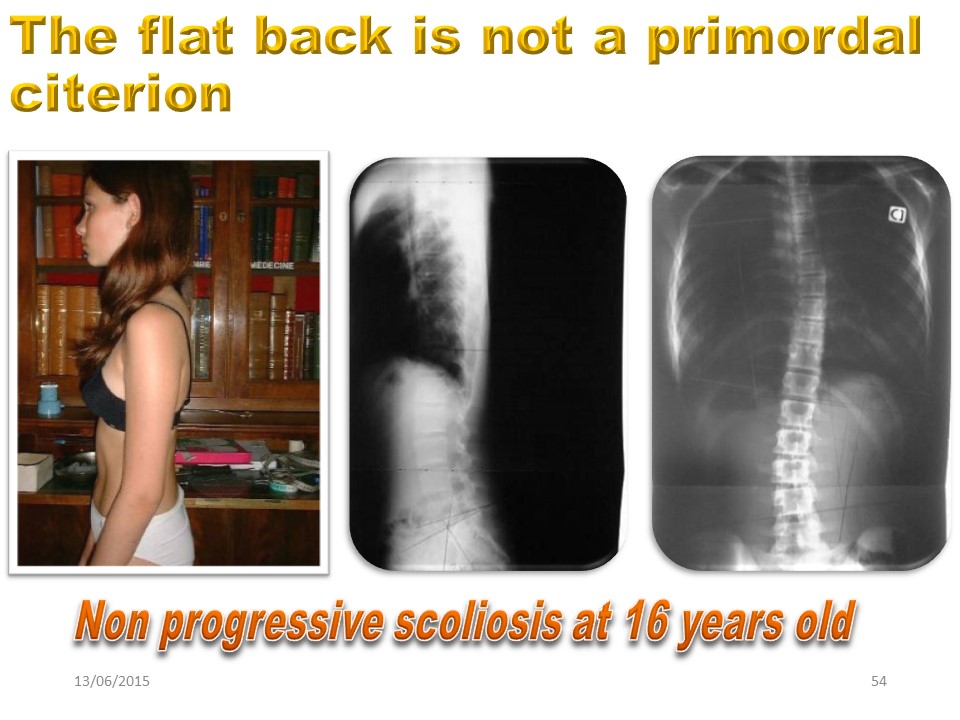 |
F or example, we often see a flat back in very active scoliosis, but the reverse is not true. This child has a large flat back, but almost no scoliosis at the end of his growth. |
 |
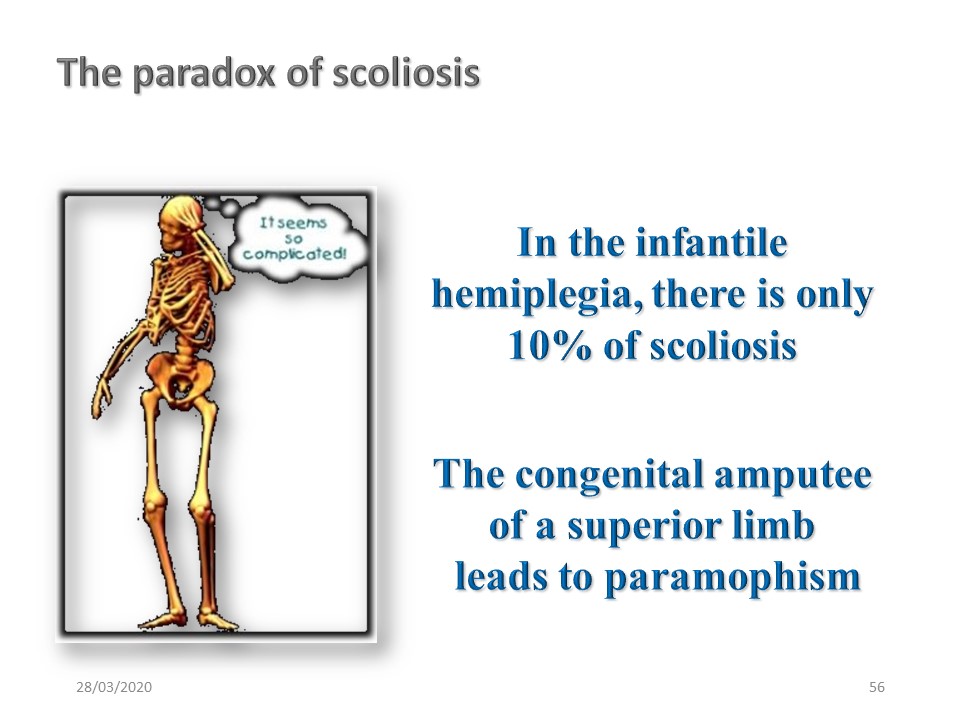
The paradox of chaos theory is that scoliosis occurs very frequently in a "normal" child. Currently, we often see progressive scoliosis with postural disorders that we have studied in the etiological chapter. But a major imbalance such as infantile hemiplegia will only cause 10% of evolutive scoliosis. |
 |
Mothers often link scoliosis with an asymmetrical posture with the school bag, which is possible in a chaotic system like the butterfly flap in Brazil, even if the structural scoliosis do not always concern children with major asymmetry. The coexistence of two chaotic and linear systems of progression is one of the characteristics of scoliosis. |
 |
The existence of thresholds is also the characteristic of scoliosis. We have mentioned the threshold of 20-25 ° for bracing, there is also a threshold of 50 ° for the surgical indication. |
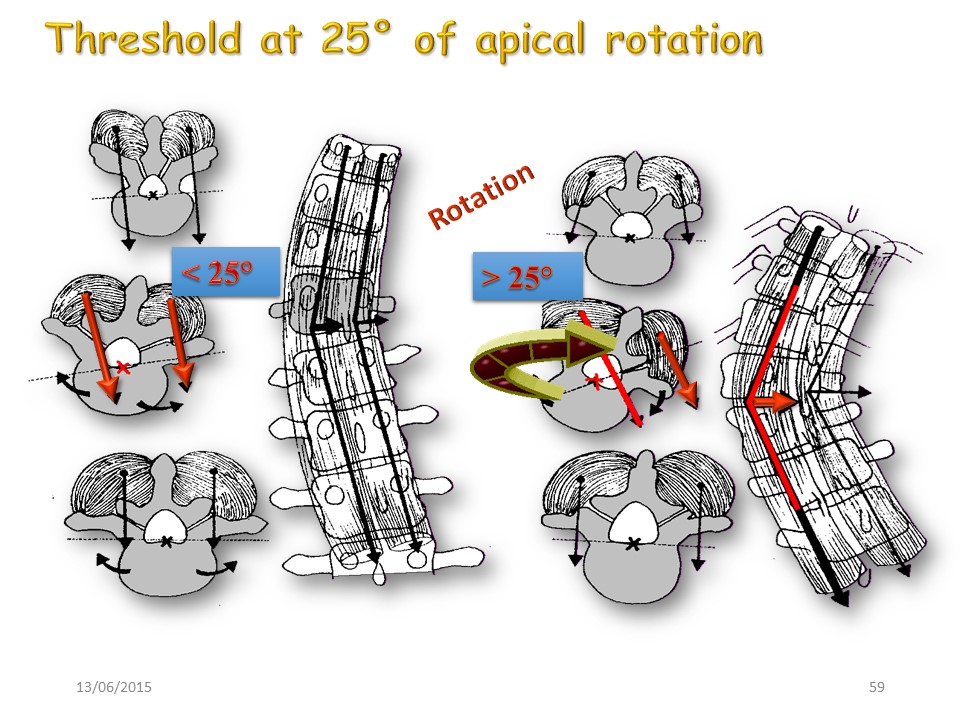 |
During an anterior flexion movement of the trunk, a weak apical rotation make it is stable, because the concave and convex muscular lever arms are located on either side of the Instant Center of Rotation (posterior wall of the vertebral body). When the rotation is greater at the apical vertebra, the lever arms are on the same side and the vertebra skids like a scooter in an excessive curve. |
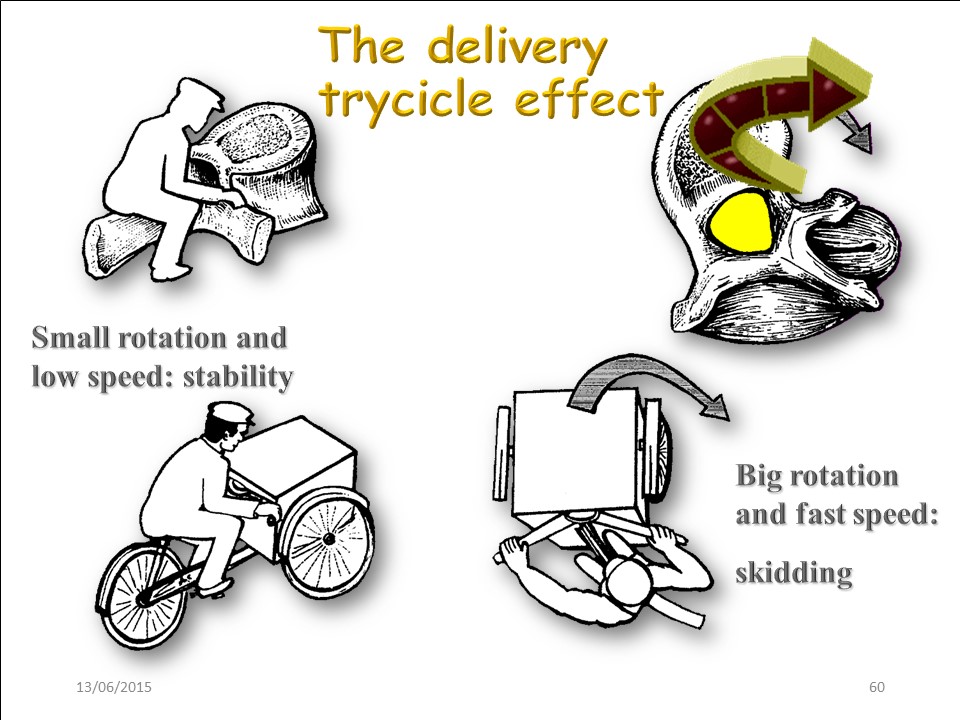 |
Besides the apical rotation, another discontinuity can occur in terms of speed. When the speed of the anterior flexion of the trunk is important, the vertebra skids like a delivery tricycle in a curve. This is why the anterior flexion movement of the trunk should be carried out slowly in scoliosis. |
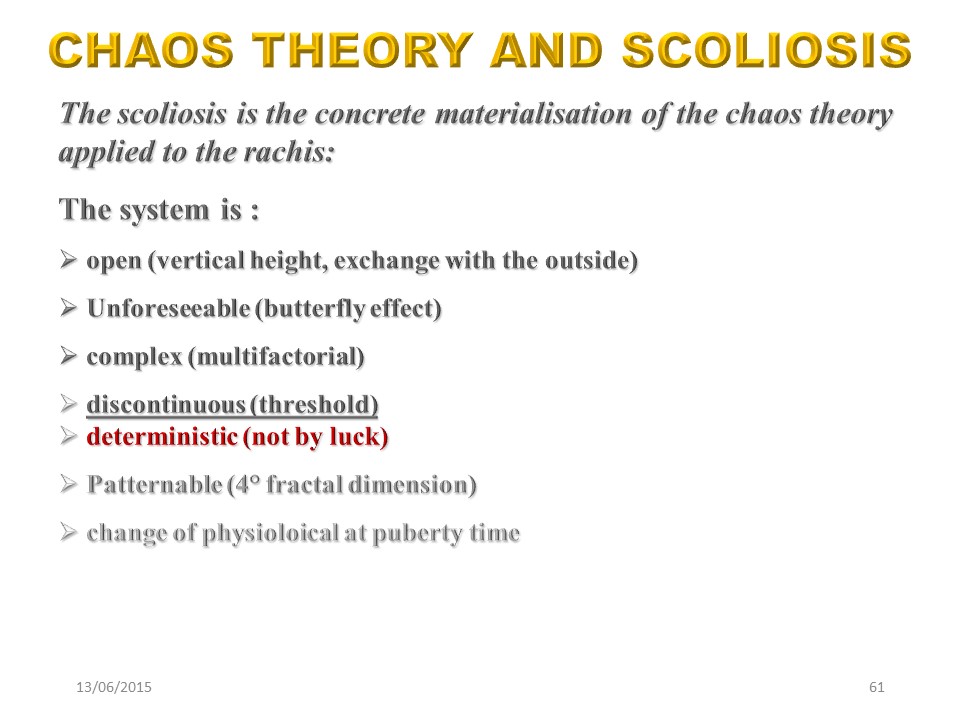 |
The chaos of scoliosis does not come at all from the laws of chance, but from complex mathematical laws, it is deterministic chaos. |
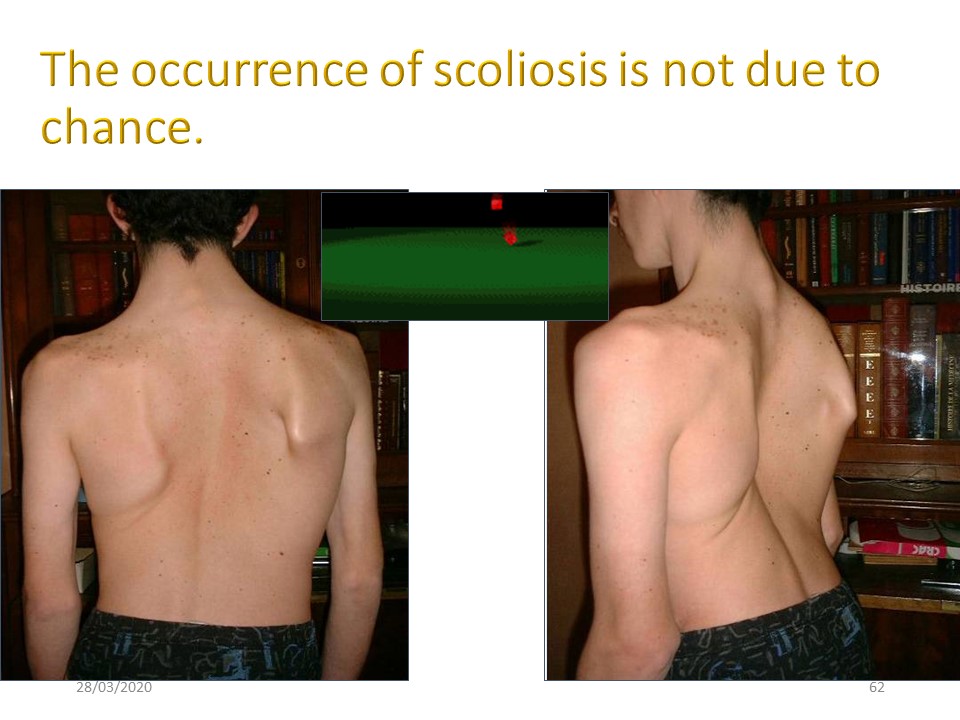 |
The occurrence of scoliosis or a flat back characteristic of scoliosis is not linked to chance. The flat back always accompanies the most progressive scoliosis, but we have seen that a flat back can exist without scoliosis. |
 |
Deterministic chaos is a concept which may define the answers to still uncovered greatest life's mysteries. In mathematics, computer science and physics, a deterministic system is a system in which no randomness is involved in the development of future states of the system. A deterministic model will thus always produce the same output from a given starting condition or initial state. the truth is that most systems in nature are chaotic. This in particular means that, even in the realm of classical biomechanics, we will in principle never be able to accurately simulate reality in which we live. However precise our measuring devices will be, we will never be able to accurately predict the behavior of the environment we live in. physics systems are deterministic because they obey deterministic differential equations. They can be conservative or dissipative. In this way, measurements made on the state of a system at a given time may not allow us to predict the future situation even moderately far ahead, despite the fact that the governing equations are exactly known. By definition, these equations are named chaotic and that they predict a deterministic chaos. |
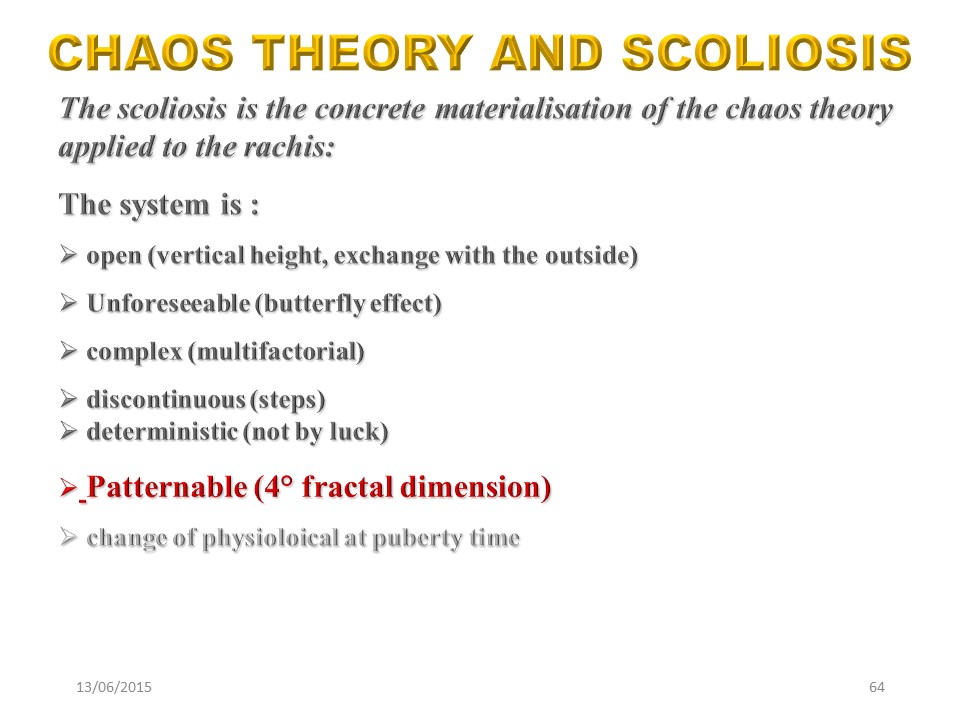 |
These equations, which are sometimes called fractals, are at the origin of the many classifications found in scoliosis and which correspond to a discontinuity. |
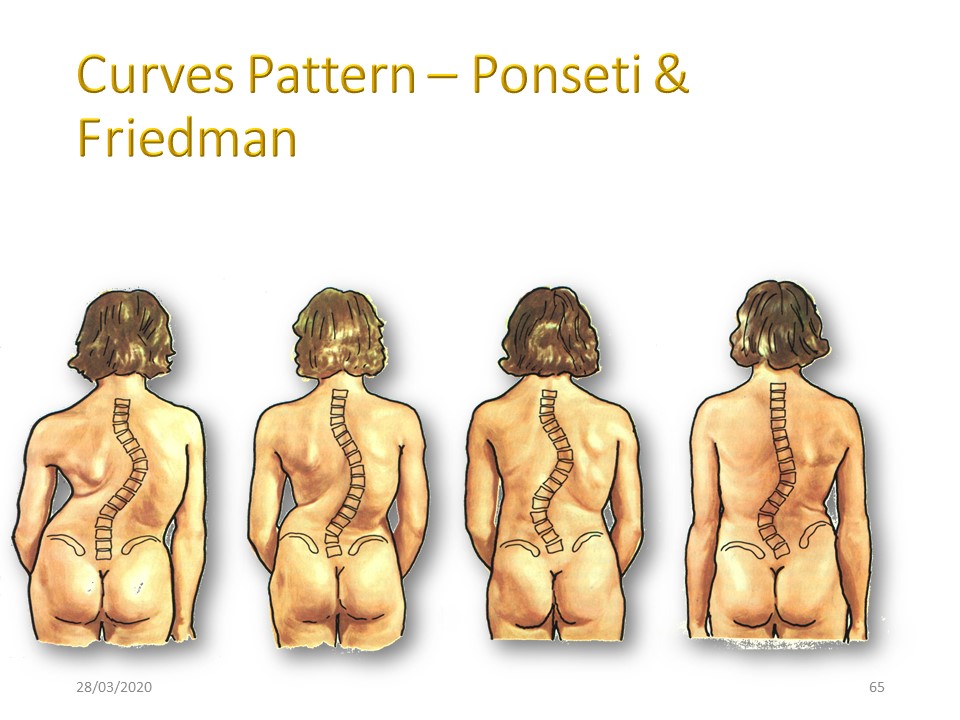 |
There are four common types of curvature in scoliosis, although lateral curves can appear anywhere along the spine. 1, the right thoracic curve. In this type, major scoliosis is concentrated in the thoracic region and curves to the right. There may also be a less severe counter-curve to the left in the lumbar region. 2, Right thoracolumbar scoliosis. The main curve is on the right in both the lower thorax and the lumbar region. This is commonly known as the C curve. 3, Right thoracic and left lumbar scoliosis. The main curve is located in the thoracic region, with an equal counter-curve on the left in the lumbar region. This is commonly known as an S curve. It looks like an S when viewed from the front. 4, Left lumbar scoliosis. The main curve is on the left in the lumbar region. There may be a less marked curve towards the right thoracic region. For unknown reasons, since lateralization at 6 years, most of the thoracic curves are on the right and most of the lumbar curves are on the left. There can be more than one compensation curve anywhere along the spine, even in the cervical spine. For childhood scoliosis before lateralization, 50% of the curvatures are on the right and 50% of the curvatures on the left. |
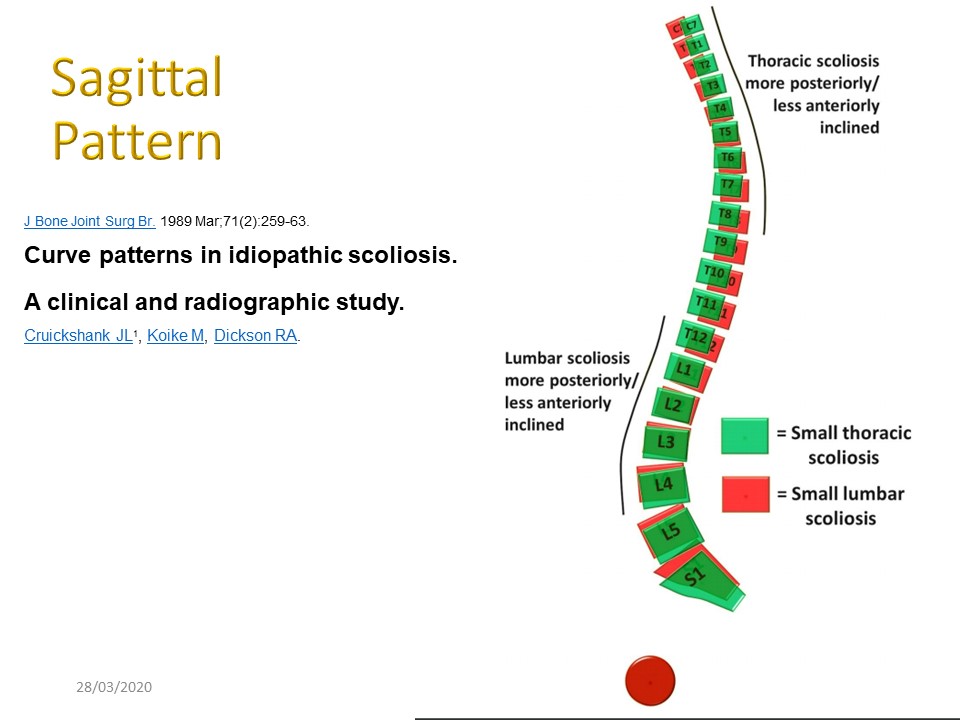 |
There are also sagittal morphotypes in scoliosis. Simple structural thoracic curves have short lordotic sections at the top, bounded above and below by kyphosis. The double curves showed longer lordotic sections limited only by a kyphosis zone. Lordosis throughout the thoracic and lumbar spine has been associated with triple curve models. There is also a difference in inclination for small thoracic scoliosis in green and small lumbar scoliosis in red. Recognition of the configuration of sagittal anomalies makes it possible to design the treatment on a solid anatomical basis for individual cases. This is possible with digital cast in three blocks. |
 |
In addition to the thoracic deformity with anterior chondro-costal projection on the side opposite the rib hump, there is also a breast asymmetry. Women with idiopathic scoliosis consistently present breast asymmetry that followed a predictable pattern. The breast on the side of the convex thoracic scoliosis curve is always smaller in volume. The affected side also presents a smaller areola, a higher position of the nipple and a higher position of the inframammary fold when compared to the opposite breast. Though the asymmetry is predictable, the degree to which the patient presents these changes does not correlate with the severity of the scoliosis. |
 |
Idiopathic scoliosis pains in adults are characteristic. The main ones were described by Winter in 1988. The convexity pain is of the muscular type, the concavity pain is of the posterior articular type. A more complete description will be detailed in the adult scoliosis module. |
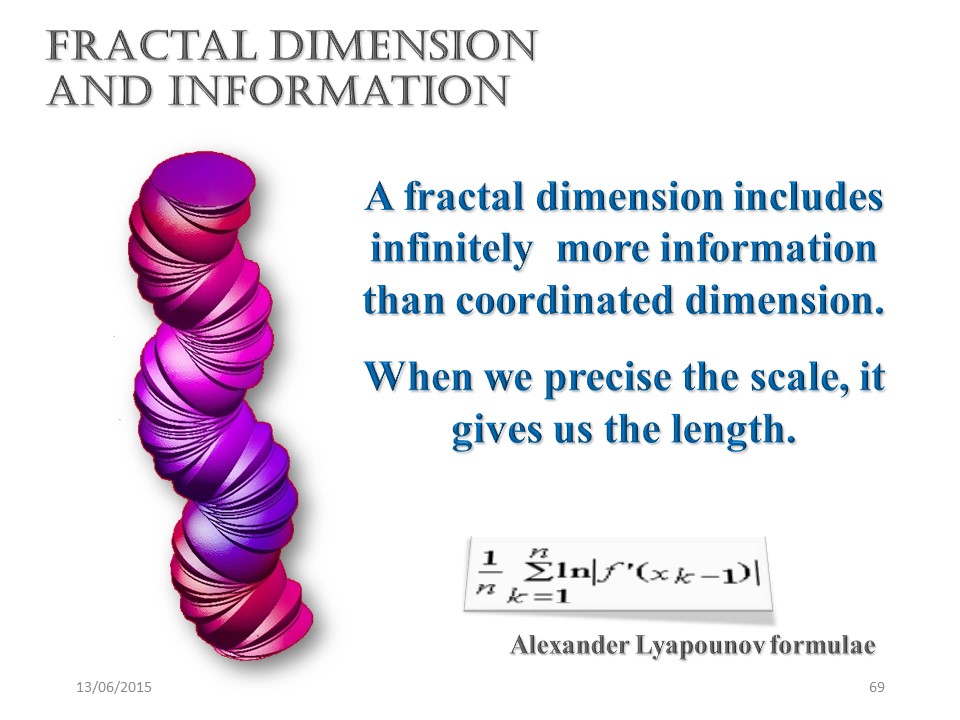 |
Scoliotic deviation can also be modelled by a fractal. A fractal dimension includes infinitely more information than coordinated dimension. When we precise the scale, it gives us the length. |
 |
The 4th dimension or the fractal dimension of deformation do not correspond to proximity laws in space neither a visible continuity. It is the appearing chaos, but in reality the causes which act in continuity one another are in a 4th dimension. Which makes for us chaos, is the visible space that has only 3 dimensions. |
 |
The Breton coast has a finite length, but it has an infinite number of lengths, each corresponding to the measurement scale that we choose. In a deformation dimension measurement system, a trajectory has an infinite number of lengths which are all summed up by a single number. |
Fibonacci is a Middle age mathematician who has introduced Arabic numerals and the zero number into Europe. The sequence found in the flower petals, starts with the numbers 0,1,1,2,3,5,8,13,21,34,55 and continues indefinitely. Two centuries later; Luca Paccioli writes "the divine proportion". This book will be illustrated by Leonardo da Vinci. We find the triangular structure that we saw in the tensegrity module and the Fibonacci spiral. His paintings are proportionate according to these Principles. In fact, there is an invisible order that mathematics allows to see. If we take an X-ray image of a human arm, it puts forward a mathematical sequence that can be found everywhere in nature. The humerus is connected to the radius and ulna of the forearm. The forearm is connected to the 8 bones of the wrist. The wrist is connected to the 5 bones of the metacarpal. The metacarpus is connected to the 3 phalanges. These numbers: 1,2,3,5,8 are part of the Fibonacci sequence. Each number is obtained by adding the two previous ones. If we transpose the numbers to the surface of squares, we obtain a perfect rectangle called a golden rectangle. If we draw an arc tangent to the perimeter of each rectangle, we obtain the Fibonacci spiral. The helical movement corresponds to that of a pine cone. This form is found in the ammonite shell. But that's not all. If we take a number of the Fibonacci sequence and divide it by the one that precedes it, we obtain a result close to 1.618, which is the number Phi called the golden number. If we take the image of the arm X-ray. The difference in length between the forearm and the hand is equal to the golden ratio. This is also valid for the 3 phalanges, but also for our DNA. The ratio between the length and the width of a complete cycle of the double helix of the DNA molecule is the golden ratio. The Fibonacci sequence and the golden ratio are examples of fractal objects. The structure of the veins of a leaf is identical to that of the branches of a tree. We have the same form repeated at infinity on an ever-smaller scale. A fractal is self-similarity. A pattern that can be seen at multiple scales of observation. Nature is based on fractal geometry because it allows it to create gigantic structures in a minimum volume. It is thanks to it, that the human body contains 96 000 km of veins and arteries. Or that 1.80 m of DNA filament can be contained in the nucleus of a cell. The totality of the DNA of a human being put end to end, we obtain a length of 6.4 billion km, a distance greater than that which separates earth from Pluto. |
|
 |
It is surprising to note that a fractal mathematical formula provides an image very close to certain organs such as the brain, the bronchi, the intestinal villi. |
 |
Chaotic phenomena occur during phase changes. |
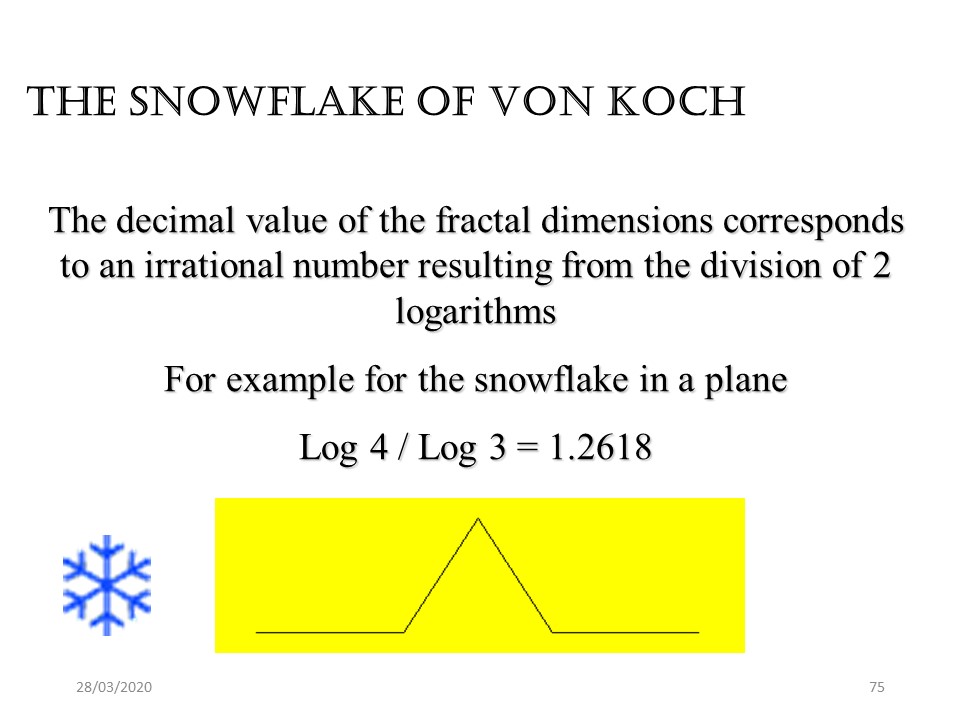 |
The snowflake which is an intermediary between liquid water and solid ice is a typical example of a chaotic structure. All snowflakes are different, but they all result from a fractal equation. The decimal value of the fractal dimensions corresponds to an irrational number resulting from the division of 2 logarithms For example for the snowflake in a plane : Log 4 / Log 3 = 1.2618 |
 |
These images mainly concern the organs which constitute an interface between gases, liquids and solids. Puberty, characteristic of homo sapiens, constitutes a sort of phase change with passage from childhood to adulthood. |
 |
In practice, this theory modifies the behavior of the doctor in terms of treatment and prevention. So-called etiological treatment of scoliosis with braces or a soleplate makes no more sense than going butterfly hunting to avoid the tornado in Texas. The chaotic system only works in one direction. Likewise, if scoliosis progresses despite physiotherapy, it will not be the physiotherapist's “fault”, any more than the earthquake-resistant construction cannot avoid the earthquake. |
 |
In the chaotic phase, earthquake-resistant construction means strengthening the extrapyramidal system without changing the structure, that is to say without seeking to correct the curvature and without readjusting the tensions. |
 |
The deformation of the railroad tracks after earthquake, that is to say after a phenomenon of elastic vibrations, reminds us that the postural system is sensitive to vibrations. |
 |
The evolution of scoliosis over 25 ° is more mechanical than postural. The extra-pyramidal system is outdated and must constantly adapt to the final deviation of the spine. |
 |
It is indeed the abnormal biomechanical phenomena that characterize this period. |
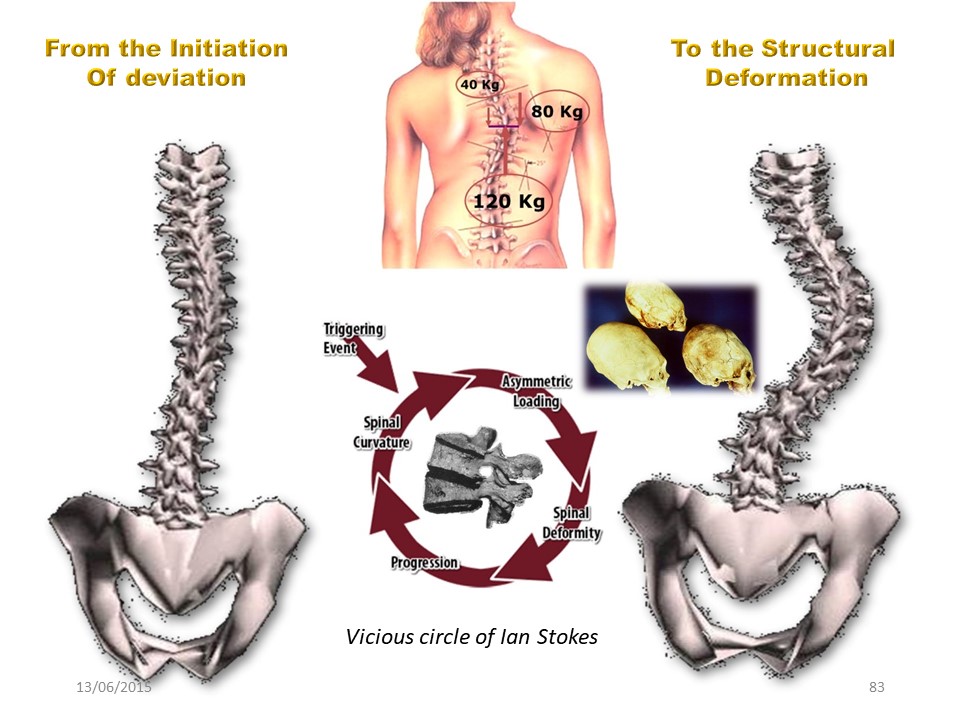 |
The initial deviation becomes structural deformation with the initiation of a biomechanical vicious circle described by Ian Stokes and accentuated by the pubertal growth of 25 cm at the level of the spine. |
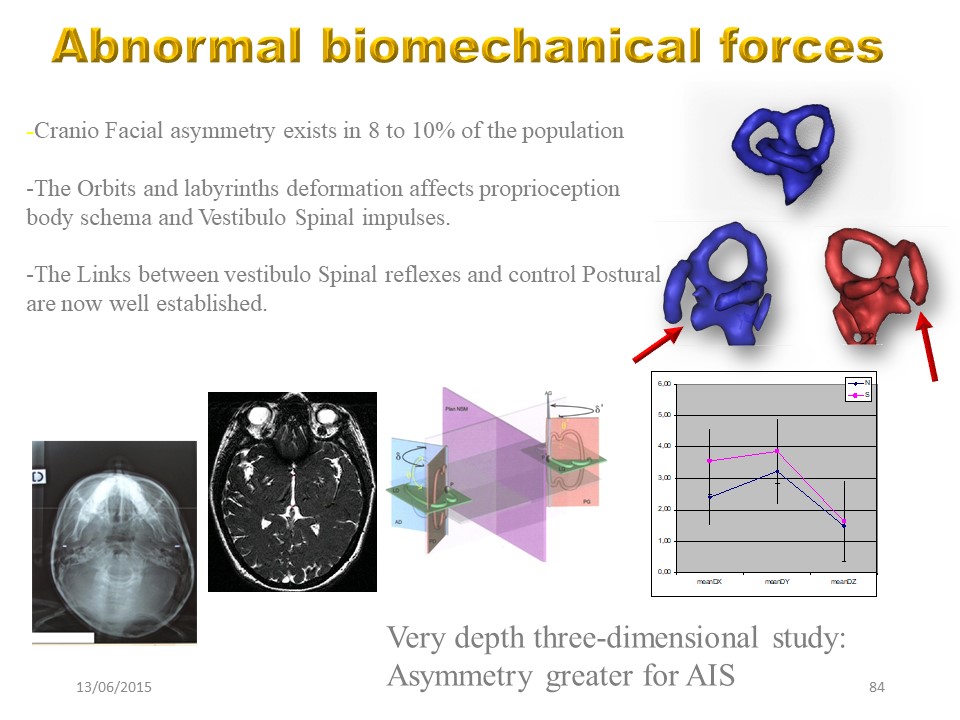 |
Abnormal biomechanical forces are associated with bone deformation, including the skull, which can modify vestibulospinal functioning. |
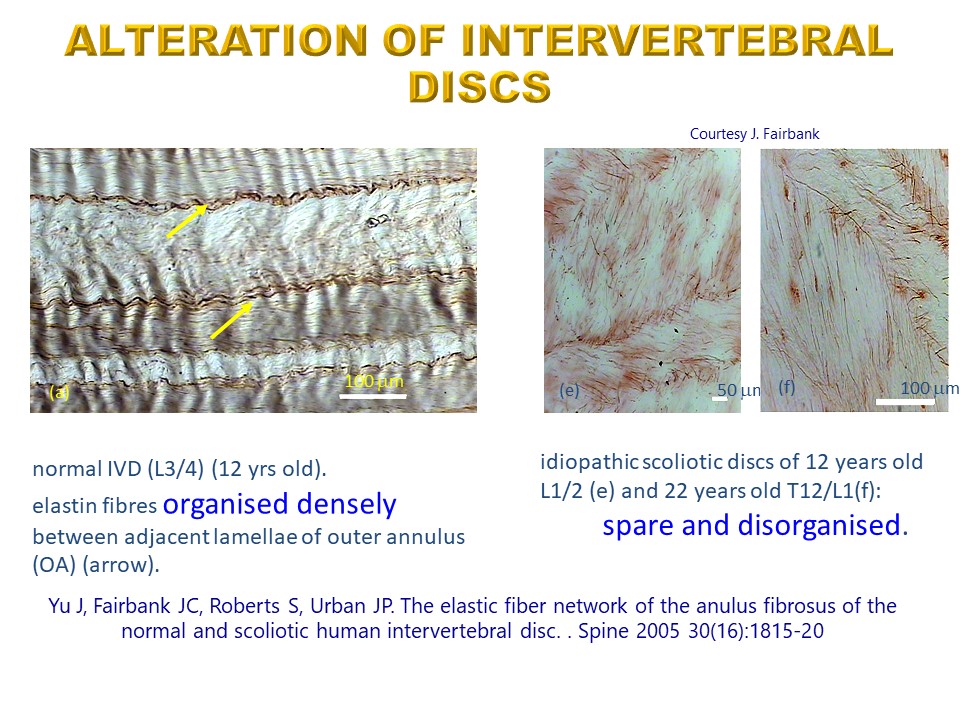 |
Abnormal biomechanical forces will also disrupt the elastic fibers inside the intervertebral disc. |
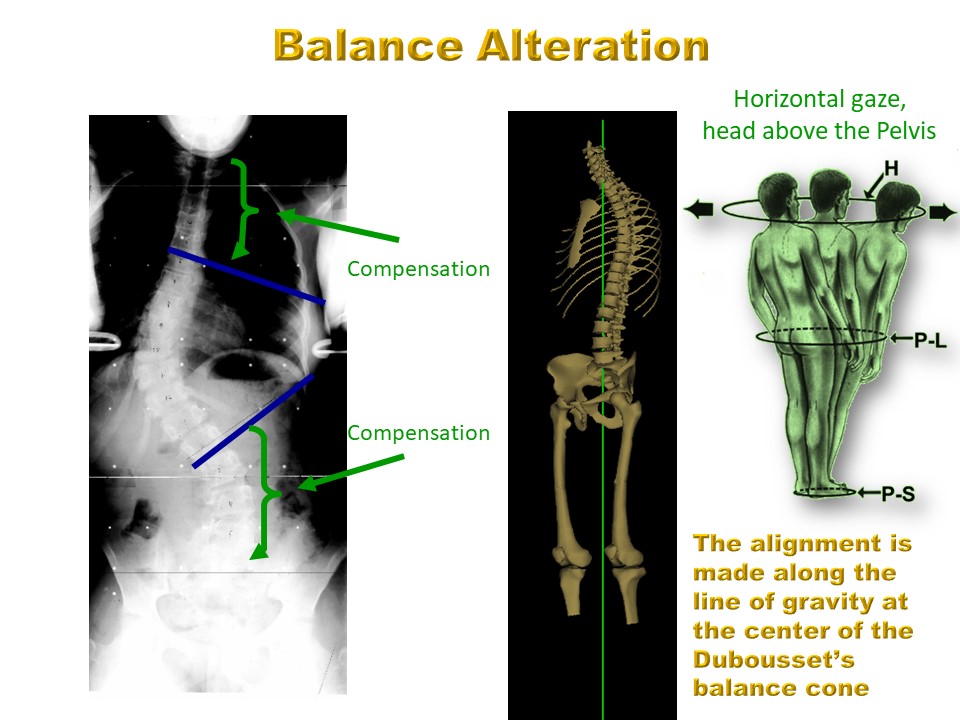 |
At this phase the rigidities linked to scoliosis can prevent the extra-pyramidal system from functioning properly, the trunk no longer being placed in its balance cone. |
 |
The deformations do not only concern the spine, but also the rib cage and the pelvis, which behaves like a vertebra. |
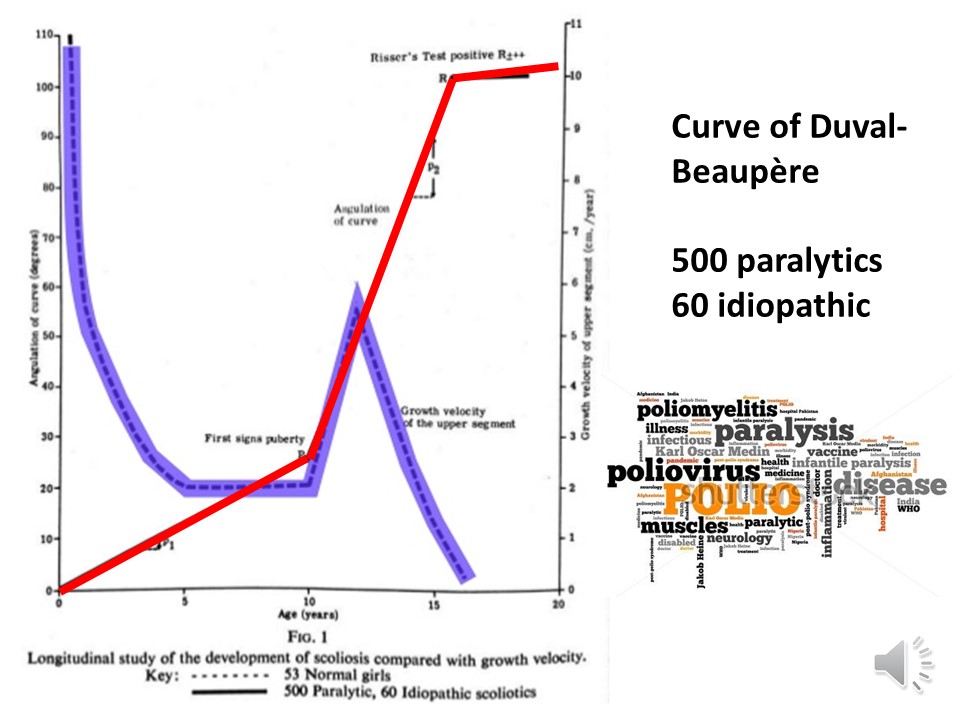 |
At this stage, all etiological considerations no longer affect the course of scoliosis. Scoliosis is considered idiopathic when there is no disease associated with scoliosis. But the etiology of scoliosis does not affect linear scoliosis as we see on the Duval-Beaupère diagram, which had been carried out initially from polio scoliosis and confirmed secondarily for idiopathic scoliosis. |
 |
Indeed, it is then a mechanical problem, with successive or simultaneous involvement of: 1. the asymmetry of paravertebral tension, 2. the asymmetrical growth of the apical vertebra. 3. the intervertebral disc asymmetry. |
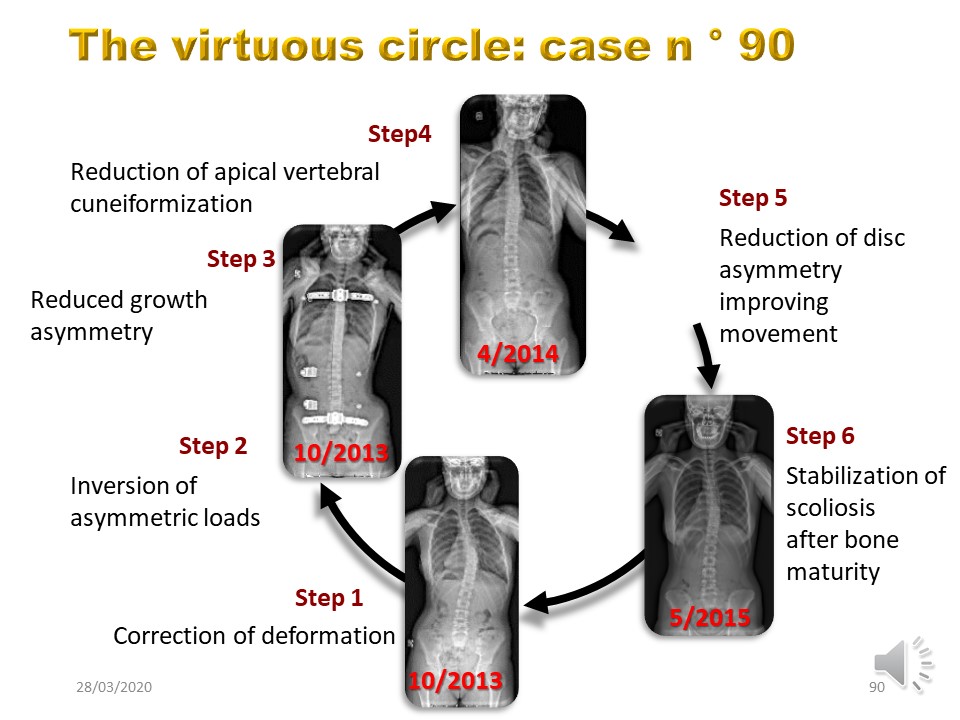 |
In order to obtain a virtuous circle, all the steps must be taken in the opposite direction: Rebalancing of the paravertebral tensions, inversion of the loads at the level of the apical vertebra, reduction of the disc asymmetry and stabilization at bone maturity. It is not only a matter of guiding growth, but of overcorrecting the curvature and maintaining the brace day and night. |
 |
The epigenetic environment is predominant during the initiation of scoliosis. Melatonin controls the majority of disturbances seen in idiopathic scoliosis. Below 20 ° of growing angulation, the evolution of scoliosis is chaotic. Physiotherapy focuses on the extra-pyramidal postural system. Beyond 20 ° of angulation during the growth period, the evolution of scoliosis is linear, and physiotherapy is primarily focused on mechanical phenomena therefore associated with the wearing of a brace. The reprogramming of the postural system must be carried out in the corrected position. |
 |
|
Free Powerpoint